Date(s) : 01/06/2023 iCal
17 h 00 min - 18 h 00 min
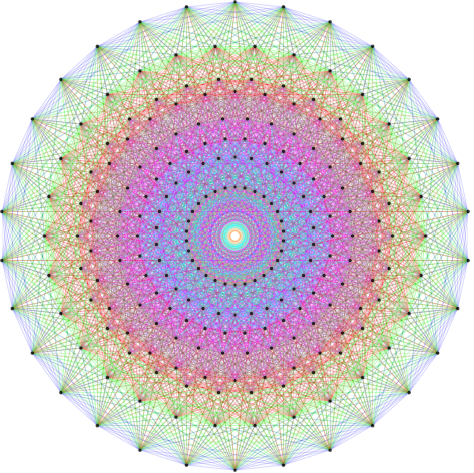
Root systems are finite sets of non-zero vectors in a Euclidean space that are subject to a list of axioms which mainly govern symmetries between them.
Originally introduced by Wilhelm Killing around 1889 in order to classify simple Lie Algebras over the field of complex numbers, root systems find applications in many mathematical areas.
In spite of their very peculiar nature, root systems have been proven to be the keystone of some of the greatest mathematical theories of the 20th century, among which we can mention the theories of Lie Algebras, Lie Groups and Algebraic Groups.
In my talk, I will try to give you a visual, and I hope accessible, introduction to root systems and to the basic concepts related to them (Weyl group / basis / positive, negative roots / coroots etc.). We will then build some fundamental root systems and give the Classification Theorem that classifies irreducible root systems thanks to a certain type of graph called Dynkin diagrams. In the end, we will give an overview of the various fields of applications of root systems in the mathematical world.
Le séminaire des doctorant⋅es de l’I2M et du CPT à Luminy regroupe chaque semaine les doctorant⋅es du site autour d’un exposé plus ou moins (in)formel, dans un cadre convivial. Il est évidemment ouvert aux étudiant⋅es de master et aux doctorant⋅es d’autres laboratoires.
C’est au rez-de-chaussée du bâtiment de « l’ancienne BU », dans la salle « Séminaire 2 » (au fond à gauche en sortant de l’escalier si vous venez de l’étage, au fond à droite si vous entrez par le rez-de-chaussée). Attention : l’entrée dans le labo nécessite un badge. En cas de souci (de type : être bloqué⋅e dehors), appeler le 04 91 26 95 91.
Emplacement
Amphi 5 - TPR2 (room 500-504, fifth floor)
Catégories