Sebastian Toth
Universität des Saarlandes, Saarbrücken
https://www.researchgate.net/profile/Sebastian-Toth
Date(s) : 27/02/2023 iCal
11 h 00 min - 12 h 00 min
For a contraction T ∈ B(H) of class C⋅0, that is SOT– limn→∞(T*)n = 0, there exists a weak-*-continuous functional calculus for H∞, the algebra of bounded holomorphic functions, first introduced by SZ.-Nagy and Foiaş. In 1986, T. Miller, R. Olin and J. Thomson proved a corresponding uniqueness statement: any continuous unital algebra homomorphism π : H∞→ B(H) with π(z) = T is weak-*-continuous and hence uniquely determined by π(z).
I will talk about a modified proof of the T. Miller, R. Olin and J. Thomson theorem. Using these modifications one can show for a large class of reproducing kernel Hilbert spaces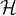 
K, including the Drury-Arveson space or the Dirichlet space on the unit ball, that the multiplier functional calculus for K-contractions, satisfying in addition a suitable C⋅0-condition, is weak-*-continuous and hence uniquely determined. This is joint work with Michael Hartz.
Emplacement
Site Nord, CMI, Salle de Séminaire R164 (1er étage)
Catégories
